The Endless Beauty of Fractals

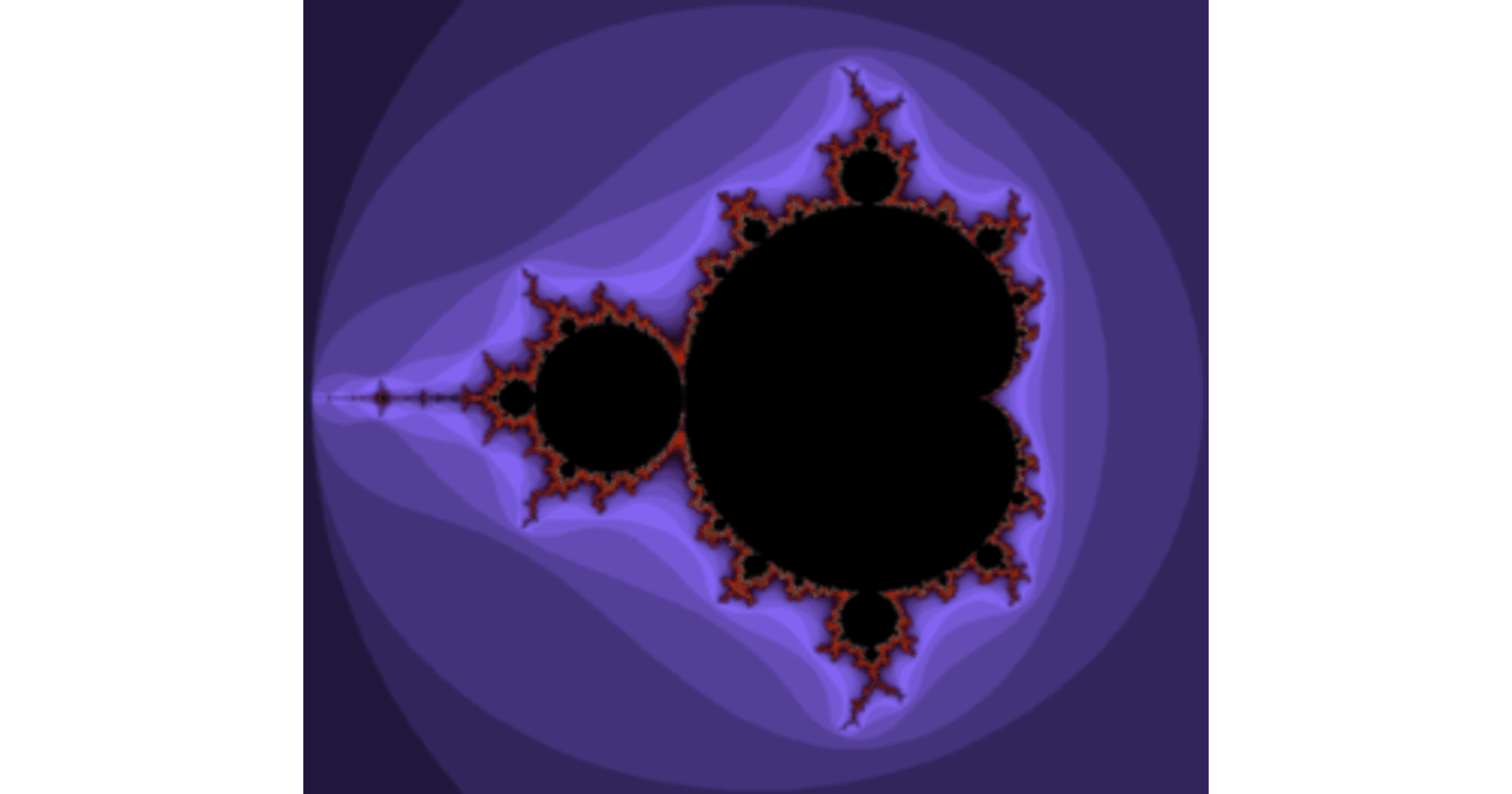
Ever heard of this astonishing phenomenon? If not, then you're in for a treat! By the end of this blog post, you'll be left speechless.😌

What are fractals?

Fractals are complex and never-ending graphical patterns that are formed by simple patterns at different scales.

The identity of a fractal lies in the fact that you can keep zooming in for eternity but still observe the same shapes as you would have seen at the start.

This concept is also known as Self-similarity.

Fractals are Everywhere...

Yes. Fractals are everywhere. It's just that you may not have recognized it.

You can find them in Nature, Geometry, Algebra, etc.

Natural Fractals: Nature's Gift

Spiral Patterns: These fractals are present in flora and fauna, swirling fluids, hurricanes as well as in galaxies.

Branching Patterns: We can find such patterns in trees, lightning strikes, rivers as well as blood vessels and neurons.

Geometrical Fractals:

Remember Pascal's Triangle used in Binomial Theorem? The numbers at the end of each row are 1 and the remaining numbers are the sum of the nearest two numbers in the above row. And this pattern keeps repeating.

Interestingly, if we colour all odd numbers with black, we get the Sierpinski Fractal Triangle!

Okay, snap out of it! Moving on 😂...

Algebraic Fractals: The Math behind fractals...

Formed by repeatedly calculating a simple equation and feeding the answer back into the equation.

One of the most famous fractals in this category is the Mandelbrot set.

This is how it works:

In the function, f(z) = z^2 + C, we put a value of C as the initial condition. Then, for each complex number z, we get f(z), which is again given as input to the function.

For some starting values of C, the function f(z) goes to infinity. For the rest, the function f(z) stays finite. These are marked by the black colour in the below picture.

Essentially, what we get is a fractal curve from the boundary of the Mandelbrot set.

Bonus! 😁

An extended version of the Mandelbrot set is the Julia set. When we increase the exponent of z in f(z) we get mesmerizing visuals!

Recap

• Fractals: Zoom in and still get the same pattern 🤯(Self-similarity)

• Natural Fractals: Spiral Patterns 😵‍💫, Branching Patterns

• Geometrical Fractals: Sierpinski Fractal Triangle

• Algebraic Fractals: Mandelbrot Set

• Bonus: Julia Set

---

That's a tad bit about the endless beauty of fractals. You can also try some fractal softwares to visualize them. Remember, this is just a drop in the ocean. But I'm sure you're in awe and won't stop exploring this!

You can reach out to me at:

Twitter | LinkedIn | Email

Until next time!